THE USE OF GENETICS IN BREEDING CHAMPIONS

 

PART 3: MENDELIAN LAWS

 

INTRODUCTION

 

The foundation is now firmly laid and we can start “building the house”. I am sure that some of you would like to comment on or criticize these articles on genetics, and you are most welcome to do so. Please don’t write me that a blue bar mated with a blue bar can only produce blue bars! This is true, as you will see, but really is not very important in breeding top quality racers.

 

Mendel published his script “Versuche über Pflanzenhybriden” (Research on Plant Hybrids) in 1866. This was the first time in history that anybody shed some light on the unexplained laws of heredity. Scientists initially either belittled or simply ignored Mendel’s work, but he insisted that his time would come. And his time did come half a century later, when his Laws were confirmed and elaborated on through other studies.

 

MENDEL’S RESEARCH

 

In his research, Mendel observed that traits were inherited as separate units, each of which was inherited independently of the others. Mendel suggested that each parent has pairs of these units but contributes only one of each pair to offspring. The units that Mendel described were later given the name genes.

 

Mendel recognised that a gene can exist in different forms. Today these alternate forms are known as alleles. For example, pea seeds have a texture trait controlled by a single gene. This gene occurs in two alleles: one corresponding to round (smooth) peas, the other to wrinkled peas. (Likewise, for example, a pigeon may have a trait for feathered feet (or not) controlled by a single gene. This gene occurs in two alleles: one corresponding to feathered feet, the other to non-feathered feet.) Although a pea (or pigeon) can carry only two alleles for a particular gene, each gene may have dozens of different alleles.

 

Mendel’s experiments focused on interbreeding different strains of pea plants and then observing the traits that appeared in subsequent generations. When he crossbred plants with round peas and those with wrinkled peas, he discovered that all of the resulting offspring had round peas. Today we know that peas are round and smooth when they contain the right amount of sugar. If peas are missing the gene that produces a protein called starch-branding enzyme (SBE1), the peas make too much sugar, causing the peas to swell and then wrinkle and shrivel as they dry.

 

Mendel concluded that when an organism has two different alleles corresponding to the same genetic traits, one of the two might be dominant. The other allele is said to be recessive, meaning that its presence will be detectable only if an organism has inherited the recessive gene from both parents. For convenience, geneticists designate alleles by a single letter – the dominant allele is represented by a capital letter and the recessive allele by a small letter. In the peas texture example, a plant inherits one allele for pea texture from each parent. The dominant allele that produces SBE1, resulting in round, smooth peas, is designated as R, while the recessive allele, that does not produce SBE1 and produces wrinkled peas is designated as r.

 

To determine the set of alleles an organism has for a given trait just by visual observation can often be difficult. In the pea plant example, for instance, plants with smooth peas might be carrying two dominant alleles for that trait (RR) or one dominant and one recessive allele (Rr). Geneticists use the term genotype to refer to the combination of genes that code for a trait, while the term phenotype describes the physical manifestation of that trait. Therefore, the presence of two dominant alleles for pea texture (RR) would reflect the genotype while a smooth pea indicates the phenotype.

 

Mendel did not limit his experiments to testing the rules of inheritance of single traits. He also studied plant traits involving multiple pairs of genes, breeding plants that have round, yellow seeds with plants that produce wrinkled, green seeds. Such experiments demonstrated that the patterns of inheritance he observed in his experiments with single traits also apply to cases involving more complex gene combinations.

 

EXCEPTIONS TO MENDELIAN LAWS

 

Mendel published his studies in a science journal in 1865, at which time no other scientist commented on his work. Since that time, geneticists have learned that sometimes genes do not easily conform to so-called Mendelian patterns of inheritance.

 

A pure dominant-recessive relationship between alleles implies that the heterozygous status cannot be distinguished from the homozygous dominant state. This is by no means the only possibility, and in fact as DNA analysis advances, it may become rare. (Bowlingsite: 4). Even without such analysis, however, there are many loci where three phenotypes (appearances) come from two alleles. But what has this to do with racing pigeons? Only this – if the same applies to pigeons, and there is no reason why it does not, applying principles of genetics to breed better racers may be rather difficult. At this early stage we can already see that using genetics to breed top class racers will require quite some initiative from the pigeon breeder.

 

MENDELIAN LAWS AND RACING PIGEONS

 

Pigeons are not plants, but the laws of heredity apply as much to them as to any other living organism. As is customary, we will use the following abbreviations in our discussion of the Mendelian Laws:

P-generation: The parents.

F1 generation: The children.

F2 generation: The grand children.

 

♂: Male pigeon.

 

♀: Female pigeon.

 

Mendel formulated three laws of heredity, namely:

Law 1: The Uniformity Law.

Law 2: The Fission Law.

Law 3: The Independence Law.

 

Law 1: The Uniformity Law. This law is also called the Law of Dominance. Two pure breeding traits brought together will produce uniform offspring in the first generation (F1 generation).

 

This means that, if two pigeons are paired, their offspring will inherit the traits that are dominant in either of the parents. For example, if a pigeon with yellow eyes are paired with a pigeon with white eyes, their offspring will have yellow eyes, because yellow is dominant over white. But it is also possible that a trait can be intermediary, i.e. is not 100% dominant.

 

So what happens when two dominant traits come together? It is known that the check colour is dominant over blue in racing pigeons, and that red is dominant over check. So if a check pigeon is paired with a red one, the offspring will be red in the F1 generation. If you are interested, the “lesser dominant” colour (check) plays what is called a hypostatic role, while the “stronger dominant” colour (red) plays an epistatic role in the transfer of traits. But remember, this only applies in the first generation (F1). In the second generation the fission law comes into play, i.e. the genes of the grandparents make things a little more complicated. (The purists in breeding for colour will probably say that it is not quite that simple – there is, for example, the issues of autosomal recessive, autosomal dominant, sex-linked recessive and sex-linked dominant which can cause different phenotypical manifestations. This is not important to us because we are not interested in breeding specific colour pigeons – our objective is to breed better quality racers! Besides, Mendel did not know about quantitative genetics.)

 

Law 2: The Fission Law. This law is also called the Law of Segregation. Simply stated it is based on the fact that genes exist in pairs in the cells of an individual, and the members of a gene pair separate into the reproductive or sex cells (also called Gametes) formed by that individual, such that half carry one member of the gene pair and half carry the other. If two pigeons that appear the same are paired, they will transfer their traits to the F2 generation (their grand children) in a particular ratio, and the chromosomes of the grand parents determine this ratio. If we were to pair the offspring of the yellow- and white eyed pigeons, their children (F2 generation) will display yellow versus white eyes in the following ratio: ¼ white, ¾ yellow. This is assuming that two of the yellow-eyed offspring will be pure yellow (homozygote) and one will be a mixture of yellow and white genes, though appearing yellow (heterozygote). (A pigeon that has matching alleles at a locus, e.g. YY or ww, is called homozygous. A pigeon that has two different alleles at a locus, e.g. Yw, is called heterozygous.)

 

Likewise, if we were to pair the two light check offspring from our second example with each other, one of their chicks will be blue and three light check.

 

How do we get to these ratios? There are three popular methods to do this. They are important, because you will use them to predict the offspring of a particular breeding cross. These methods are:

1.                  The Punnett Square.

2.                  The Branching System.

3.                  The FOIL Method.

 

The Punnet Square. The Punnett Square can be used to reflect the combination of any characteristics, but we will use the homozygous yellow (dominant) vs white (recessive) eye colour as an example.

 

		♂ (Eyes appear yellow – impure)
		YELLOW	white
♀ (Eyes appear yellow – impure)	YELLOW	YELLOW/ YELLOW (pure yellow eyes)	YELLOW/ White (impure yellow eyes)
	White	white/ YELLOW (impure yellow eyes)	white/ white (pure white eyes)

 

Figure 4: The Punnet Square.

 

The Punnett Square can be expanded to reflect more than one trait. However, this method becomes very cumbersome when dealing with more than two different traits. The following is an example of a dihybrid cross (two traits simultaneously). The example will also be used to illustrate how one can calculate the phenotypic ratio. Let’s use eye colour (Y = yellow and w = white) as well as feather colour (R = red and b = blue bar, with blue bar being recessive compared to red, which is, therefore, dominant.)

 

	Male (♂)
Female (♀)		YR	Yb	wR	wb
	YR	YR, YR	Yb, YR	wR,YR	wb, YR
	Yb	YR, Yb	Yb, Yb	wR, Yb	wb, Yb
	wR	YR, wR	Yb, wR	wR, wR	wb, wR
	wb	YR, wb	Yb, wc	wR, wb	wb, wb

 

GENOTYPE	GENOTYPE RATIO	GENOTYPE	GENOTYPE RATIO
YR, YR	1/16	YR, wR	1/16
Yb, YR	1/16	Yb, wR	1/16
wR,YR	1/16	wR, wR	1/16
wb, YR	1/16	wb, wR	1/16
YR, Yb	1/16	YR, wb	1/16
Yb, Yb	1/16	Yb, wb	1/16
wR, Yb	1/16	wR, wb	1/16
wb, Yb	1/16	wb, wb	1/16

 

PHENOTYPE	PENOTYPE DESCRIPTION	PHENOTYPE RATIO
YR, YR	Yellow eyes, red feather colour.	9/16
Yb, YR
wR,YR
wb, YR
YR, Yb
wR, Yb
YR, wR
Yb, wR
YR, wb
Yb, Yb	Yellow eyes, blue bar feather colour.	3/16
wb, Yb
Yb, wb
wb, wb	White eyes, blue bar feather colour.	1/16
wR, wR	White eyes, red feather colour.	3/16
wb, wR
wR, wb

 

The branch Systems Diagram. Another way to illustrate the results of mating is by means of a Branch Systems Diagram. The following is an example of a Branch Systems Diagram:

 

Figure 5: The Branch Systems Diagram.

 

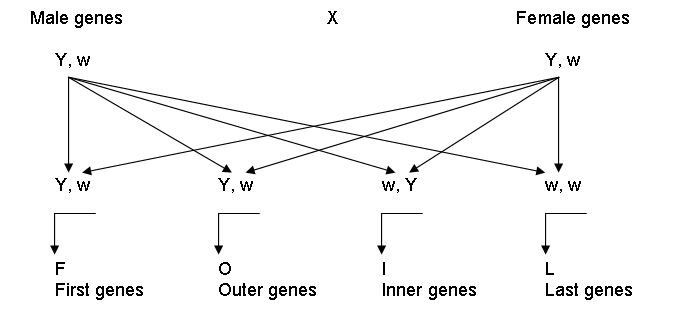
The FOIL Method. A third method to illustrate the results of mating is by means of the FOIL Method. The FOIL Method comes from the algebraic expression (a+b)(c+d) = ac+ad+bc+bd. FOIL comes from the four steps followed in this method:

1.                  Combine the first (F) of each pair.

2.                  Combine the outer (O) of each pair.

3.                  Combine the inner (I) of each pair.

4.                  Combine the last (L) of each pair.

 

This can be illustrated as follows:

 

  

 

Figure 6: The FOIL Method.

 

The FOIL method is easy to use, but from a presentation standpoint does not lend itself very well to showing the sequence for how the outcome was derived. It can only be applied to a cross involving a single pair of alleles. If this method is used for crosses involving more than one trait then the individual gene pairs of the first cross need to be combined with the individual gene pairs of the second cross to show all possible outcomes.

 

I will use a simplified combination of the Branch System and FOIL Method to illustrate arguments in these articles. The following is an example:

 

 

                                                                                               

 

 

 

 

 

 

 

 

           

 

 

 

 

 Figure 7: The combination method.

 

Law 3: The Independence Law. Also called the Law of Independent Assortment. This law explains the behaviour of genes controlling separate traits. The genes controlling separate traits segregate independently; that is, segregation at one locus does not influence segregation at another.

 

When paring two pigeons (P-generation) that differ in more than one trait, this (differing) trait will be passed on independently. Take, for example, a blue bar cock with yellow eyes paired to a pure check hen with white eyes. In the F1 generation we will find that:

•            The yellow eyes will dominate over the white eyes, so the offspring will have yellow eyes.

•            The check colour will be passed on intermediary, i.e. the offspring will be light check in colour.

 

So, if we now pair the yellow-eyed light check offspring with one another, we will get the following in the F2 generation:

•          Three of the four will be checks, of which 75% will have yellow eyes and 25% will have white eyes.

•          One of the four will be a blue bar, of which 75% will have yellow eyes and 25% will have white eyes.

 

The observant reader will now already wonder how many pigeons two combinations of 75% and 25% represent if only four offspring are involved. This demonstrates that we are working with probabilities and not exact numbers. So, theoretically, if we were to breed 16 chicks from the yellow-eyed light checks, 12 will be checks, of which 9 will have yellow eyes and 3 will have white eyes. Of the four blue bars three will have yellow eyes and one will have white eyes. But which ones in each batch of two chicks will be checks or blue bars and which of them will have yellow or white eyes? As I see it this is much like flipping a coin. Statistically, when you flip a coin it should land heads (or tails) 50% of the time. Try this and you will see that it does not work that way. If you flip the coin ten times, chances are good that it will not land heads and tails five times each. But the more times you flip the coin, the closer you will get to a ratio of 50/50. Although Mendel’s Laws have a precise mathematical basis, the randomness of segregation and recombination may lead by chance to unexpected frequencies of phenotypes.

 

Fine, now we have some explanation for the ratio issue. But this is only one issue that makes the use and control of genetics in breeding rather complicated. Next time we will look at some others.

 

Dr Jaap Nel. Tel no (012) 653 2119. Fax no: (012) 653 7030. Email: jaap7@iafrica.com.